
He has been the CFO or controller of both small and medium sized companies and has run small businesses of his own. He has been a manager and an auditor with Deloitte, a big 4 accountancy firm, and holds a degree from Loughborough University. In the present value formula shown above, we’re assuming that you know the future value and are solving for present value. A financial professional will offer guidance based on the information provided and offer a no-obligation call to better understand your situation. For information pertaining to the registration status of 11 Financial, please contact the state securities regulators for those states in which 11 Financial maintains a registration filing. 11 Financial may only transact business in those states in which it is registered, or qualifies for an exemption or exclusion from registration requirements.
Present Value of a Perpetuity (t → ∞) and Continuous Compounding (m → ∞)

Get instant access to video lessons taught by experienced investment bankers. Learn financial statement modeling, DCF, M&A, LBO, Comps and Excel shortcuts. Given a higher discount rate, the implied present value will be lower (and vice versa). If you expect to have $50,000 in your bank account 10 years from now, with the interest rate at 5%, you can figure out the amount that would be invested today to achieve this. One key point to remember for PV formulas is that any money paid out (outflows) should be a negative number, while money in (inflows) is a positive number. My course, Expectancy Wealth Planning, has been called „the best financial education on the internet“ and provides all the knowledge you’ll ever need to build the life — and retirement — of your dreams.
Other important present value calculations
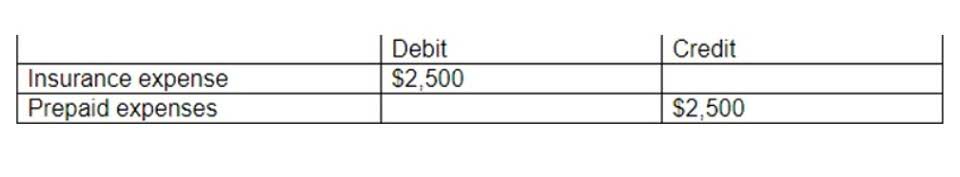
This means that the future value problem involves compounding while present value problems involve discounting. For example, if your payment for the PV formula is made monthly, then you’ll need to convert your annual interest rate to monthly by dividing by 12. Also, for NPER, which pv of single sum table is the number of periods, if you’re collecting an annuity payment monthly for four years, the NPER is 12 times 4, or 48. Present value factor (PVF) (also called present value interest factor (PVIF)) is the equivalent value today of $1 in future or a series of $1 in future.
What’s in the Present Value Calculation
To illustrate, let’s assume that $1,000 will be invested today at an annual interest rate of 8% compounded annually. Because we know three components, we can solve for the unknown fourth component—the number of years it will take for $1,000 of present value to reach the future value of $5,000. We’ll discuss PV calculations that solve for the present value, the implicit interest rate, and/or the length of time between the present and future amounts. A present value of 1 table states the present value discount rates that are used for various combinations of interest rates and time periods.
This is because money today tends to have greater purchasing power than the same amount of money in the future. Taking the same logic in the other direction, future value (FV) takes the value of money today and projects what its buying power would be at some point in the future. The first column (n) refers to the number of recurring identical payments (or periods) in an annuity. The other columns contain the factors for the interest rate (i) specified in the column heading. The point where a particular interest rate (i) intersects a particular number of payments (n) is the annuity’s PVOA factor. When you multiply this factor by the annuity’s recurring payment amount, the result is the present value of the annuity.

Because the PV of 1 table had the factors rounded to three decimal places, the answer ($85.70) differs slightly from the amount calculated using the PV formula ($85.73). Present value of a future single sum of money is the value that is obtained when the future value is discounted at a specific given rate of interest. Let’s assume we have a series of equal present values that we will call payments (PMT) for n periods at a constant interest rate i. We can calculate FV of the series of payments 1 through n using formula (1) to add up the individual future values. Present value is important in order to price assets or investments today that will be sold in the future, or which have returns or cash flows that will be paid in the future.
Present value (PV) is the current value of a future sum of money or stream of cash flows. It is determined by discounting the future value by the estimated rate of return that the money could earn if invested. Present value calculations can be useful in investing and in strategic planning for businesses. If you don’t have access to an electronic financial calculator or software, an easy way to calculate present value amounts is to use present value tables (PV tables). PV tables cannot provide the same level of accuracy as financial calculators or computer software because they use factors that are rounded off to fewer decimal places.
- Present value (PV) is the current value of an expected future stream of cash flow.
- Our online tools will provide quick answers to your calculation and conversion needs.
- Both (n) and (i) are stated within the context of time (e.g., two years at a 10% annual interest rate).
- The sum of all the discounted FCFs amounts to $4,800, which is how much this five-year stream of cash flows is worth today.
- The word „discount“ refers to future value being discounted back to present value.
Because transactions take place in the present, those future cash flows or returns must be considered by using the value of today’s money. To calculate the present value of a stream of future cash flows you would repeat the formula for each cash flow and then total them. Fortunately, you can easily do this using software or an online calculator rather than by hand.

This is equivalent to saying that at a 12% interest rate compounded annually, it does not matter whether you receive $8,511.40 today or $15,000 at the end of 5 years. In present value situations, the interest rate is often called the discount rate. Some individuals refer to present value problems as „discounted present value problems.“ Calculate the present value of this sum if the current market interest rate is 12% and the interest is compounded annually. For example, suppose you want to know the value today of receiving $15,000 at the end of 5 years if a rate of return of 12% is earned. Many times in business and life, we want to determine the value today of receiving a specific single amount at some time in the future.
(Discounting means removing the interest that is imbedded in the future cash amounts.) As a result, present value calculations are often referred to as a discounted cash flow technique. We need to calculate the present value (the value at time period 0) of receiving a single amount of $1,000 in 20 years. The interest rate for discounting the future amount is estimated at 10% per year compounded annually. A mentioned, the discount rate is the rate of return you use in the present value calculation.
